Extra Questions: Class 9 Maths Chapter 2 Polynomials
NCERT Class 9 Maths Chapter 2 Polynomials :
- NCERT Class 9 Maths Chapter 2 Polynomials Exercise 2.1
- NCERT Class 9 Maths Chapter 2 Polynomials Exercise 2.2
- NCERT Class 9 Maths Chapter 2 Polynomials Exercise 2.3
- NCERT Class 9 Maths Chapter 2 Polynomials Exercise 2.4
- NCERT Class 9 Maths Chapter 2 Polynomials Exercise 2.5
- Extra Questions Class 9 Maths Chapter 2 Polynomials
Question 1. Which of the following expressions are polynomials?
(i) x5 - 2x3 + + x + 7
Solution :
We have x5 - 2x3 + + x + 7
The highest degree of the variable = 5
(ii) \(\displaystyle \frac{1}{{\sqrt{2}}}{{x}^{2}}\,-\,\sqrt{2}x\,+\,2\)
Solution :
We have \(\displaystyle \frac{1}{{\sqrt{2}}}{{x}^{2}}\,-\,\sqrt{2}x\,+\,2\)
The highest degree of the variable = 2
Question 2. Coefficient of x in √3 - 2√2x + 4x2
Solution :
We have √3 - 2√2x + 4x2
The coefficient of x is 2√2x
Question 3. Classify the following as linear, quadratic, and cubic polynomials:
(i) 2x2 + 4x
Solution :
We have 2x2 + 4x
It is a quadratic polynomial.
Question 4. If p(x) = 5 - 4x + 2x2 , find (i) p(0) (ii) p(3) (iii) p(-2)
Solution :
Given 5 - 4x + 2x2
[ After put x = 0 ]
5 - 4(0) + 2(0)2
= 5 - 0 + 2 × 0
= 5 - 0 + 0
= 5
[ After put x = 3 ]
5 - 4(3) + 2(3)2
= 5 - 12 + 2 × 9
= 5 - 12 + 18
= 23 - 12
= 11
[ After put x = -2 ]
5 - 4(-2) + 2(-2)2
= 5 + 8 + 2 × 4
= 5 + 8 + 8
= 21
After put p(0), p(3) and p(-2) we get 5, 11 and 21.
Question 5. Find the zero of the polynomial:
(i) p(x) = x - 5
Solution :
Given x - 5
x - 5 = 0
= x = 5
(ii) g(x) = 5 - 4x
Given 5 - 4x
5 - 4x = 0
= - 4x = - 5
= x = \(\displaystyle \frac{{-\,5}}{{-\,4}}\)
= x = 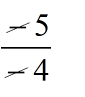
= x = \(\displaystyle \frac{{\,5}}{4}\)
Question 6. Using remainder theorem, find the remainder when.
(i) ( x3 - 6x2 + 9x + 3 ) is divided by ( x - 1 )
Solution :
Let p(x) = x3 - 6x2 + 9x + 3
So, x - 1 = 0
x = 1
Using remainder theorem,
when p(x) = x3 - 6x2 + 9x + 3
than p ( 1 ) = ( 1 )3 - 6( 1 )2 + 9 × 1 + 3
= 1 - 6 × 1 + 9 + 3
= 1 - 6 + 9 + 3
= 13 - 6
= 7
So, answer = 7
Question 7. Using factor theorem, show that:
(i) ( x - 2 ) is a factor of ( x3 - 8 )
Solution :
Let p(x) = x3 - 8
So, x - 2 = 0
x = 2
Using remainder theorem,
when p(x) = x3 - 8
than p ( 2 ) = ( 2 )3 - 8
= 8 - 8
= 0
So, p(x) is a factor of ( x3 - 8 )
Question 8. Find the value of k for which ( x - 1 ) is a factor of ( 2x3 + 9x2 + x + k )
Solution :
Let p(x) = 2x3 + 9x2 + x + k
Given x - 1 is factor of p(x)
So, x - 1 = 0
x = 1
Using the factor theorem,
when p( x ) = 2x3 + 9x2 + x + k
than p(1) = 2(1)3 + 9(1)2 + 1 + k
= 2 × 1 + 9 × 1 + 1 + k = 0
= 2 + 9 + 1 + k = 0
= 12k = 0
= k = -12
The value of k = -12
Question 9. Factorize :
(i) 18x2y - 24xyz
Solution :
Given 18x2y - 24xyz
18x2y - 24xyz [ find the common ]
= 6xy ( 3x - 4z )
So, answer = 6xy ( 3x - 4z )
(ii) x3 - 2x2y + 3xy2 - 6y3
Solution :
Given x3 - 2x2y + 3xy2 - 6y3
x3 - 2x2y + 3xy2 - 6y3 [ find the common ]
= x2 ( x - 2y ) + 3y2 ( x - 2y )
= ( x2 + 3y2 ) ( x - 2y )
So, answer = ( x2 + 3y2 ) ( x - 2y )
Question 10. Factorize :
(i) 25x2 - 64y2
Solution :
Given 25x2 - 64y2
[ Using identity = ( a + b ) ( a - b ) = a2 - b2 ]
25x2 - 64y2
= ( 5x + 8y ) ( 5x - 8y )
∴ 25x2 - 64y2 = ( 5x + 8y ) ( 5x - 8y )
(ii) ( 4x - 2y )3
Solution :
We have ( 4x - 2y )3
[ using identity = ( x - y )3 = x3 - y3 - 3xy ( x - y ) ]
( 4x - 2y )3
= ( 4x )3 - ( 2y )3 - 3 × 4x × 2y ( 4x - 2y )
= 64x3 - 8y3 - 24xy ( 4x - 2y )
= 64x3 - 8y3 - 96x2y + 48xy2
So, answer = 64x3 - 8y3 - 96x2y + 48xy2
Question 11. Factorize :
(i) x2 + 18x + 32
Solution :
Given x2 + 18x + 32
[ Using the splitting the middle term method ]
So, x2 + 18x + 32
= x2 + 16x + 2x + 32 [ find the common ]
= x ( x + 16 ) + 2 ( x + 16 )
= ( x + 2 ) ( x + 16 )
(ii) 7x + 49x + 84
Solution :
Given 7x + 49x + 84
[ Using the splitting the middle term method ]
So, 7x + 49x + 84
= 7x + 28x + 21x + 84
= 7x ( 1 + 4 ) + 1x ( 1 + 4 )
= ( 7x + x ) ( 1 + 4 )
(iii) √2x2 + 3x + √2
Solution :
Given √2x2 + 3x + √2
[ Using the splitting the middle term method ]
√2x2 + 2x + x + √2
= √2x ( x + √2 ) + 1 ( x + √2 )
= ( x + √2 ) ( √2x + 1 )
Question 12. Expand :
(i) ( a + 2b + 5c )2
Solution :
We have ( a + 2b + 5c )2
[ using identity = ( x + y + z )2 = ( x2 + y2 + z2 + 2xy + 2yz + 2zx ) ]
( a + 2b + 5c )2
= ( a )2 + ( 2b )2 + ( 5c )2 + 2 × a × 2b + 2 × 2b × 5c + 2 × 5c × a
= a2 + 4b2 + 25c2 + 4ab + 20bc + 10ac
So, answer = a2 + 4b2 + 25c2 + 4ab + 20bc + 10ac
(ii) ( a - 2b - 3c )2
Solution :
We have ( a - 2b - 3c )2
[ using identity = ( x + y + z )2 = ( x2 + y2 + z2 + 2xy + 2yz + 2zx ) ]
( a - 2b - 3c )2
= ( a )2 + ( -2b )2 + ( -3c )2 + 2 × a × ( -2b ) + 2 × ( -2b ) × ( -3c ) + 2 × ( -3c ) × a
= a2 + 4b2 + 9c2 - 4ab + 12bc - 6ac
So, answer = a2 + 4b2 + 9c2 - 4ab + 12bc - 6ac
Question 13. Factorize :
(i) 9x2 + 16y2 + 4z2 - 24xy + 16yz - 12xz
Solution :
We have 9x2 + 16y2 + 4z2 - 24xy + 16yz - 12xz
[ using identity = ( x + y + z )2 = ( x2 + y2 + z2 + 2xy + 2yz + 2zx ) ]
9x2 + 16y2 + 4z2 - 24xy + 16yz - 12xz
= ( -3x)2 + ( 4y )2 + ( 2z )2 + 2 × ( -3x ) × 4y + 2 × 4y × 2z + 2 × 2z × ( -3x )
= ( -3x + 4y + 2z )2
= ( -3x + 4y + 2z ) ( -3x + 4y + 2z )
So, answer = ( -3x + 4y + 2z ) ( -3x + 4y + 2z )
(ii) 4x2 + 9y2 + 16z2 + 12xy - 24yz - 16xz
Solution :
We have 4x2 + 9y2 + 16z2 + 12xy - 24yz - 16xz
[ using identity = ( x + y + z )2 = ( x2 + y2 + z2 + 2xy + 2yz + 2zx ) ]
4x2 + 9y2 + 16z2 + 12xy - 24yz - 16xz
= ( 2x )2 + ( 3y )2 + ( -4z )2 + 2 × 2x × 3y + 2 × 3y × ( -4z ) + 2 × ( -4z ) × 2x
= ( 2x + 3y - 4z )2
= ( 2x + 3y - 4z ) ( 2x + 3y - 4z )
So, answer = ( 2x + 3y - 4z ) ( 2x + 3y - 4z )
Question 14. Evaluate :
(i) ( 99 )2
Solution :
We have ( 99 )2
( 100 - 1 )2
[ using identity = ( x - y )2 = x2 - 2xy + y2 ]
( 100 - 1 )2
= ( 100 )2 - 2 × 100 × 1 + ( 1 )2
= 10000 - 200 + 1
= 10001 - 200
= 9801
So, answer = 9801
(ii) 991 × 1009
Solution :
We have 991 × 1009
( 1000 - 9 ) × ( 1000 + 9 )
[ using identity = x2 - y2 = ( x + y ) ( x - y) ]
( 1000 - 9 ) × ( 1000 + 9 )
= ( 1000 )2 - ( 9 )2
= 1000000 - 81
= 999919
So, answer = 999919
(iii) 117 × 83
Solution :
We have 117 × 83
( 100 + 17 ) × ( 100 - 17 )
[ using identity = x2 - y2 = ( x + y ) ( x - y) ]
( 100 + 17 ) × ( 100 - 17 )
= ( 100 )2 - ( 17 )2
= 10000 - 289
= 9711
So, answer = 9711
Question 15. Expand :
(i) ( \(\displaystyle \frac{2}{3}x\) + 1 )3
Solution :
We have ( \(\displaystyle \frac{2}{3}x\) + 1 )3
[ using identity = ( x + y )3 = x3 + y3 + 3xy ( x + y ) ]
( \(\displaystyle \frac{2}{3}x\) + 1 )3
= \(\displaystyle {{\left( {\frac{2}{3}x} \right)}^{3}}\) + ( 1 )3 + 3 × \(\displaystyle \left( {\frac{2}{3}x} \right)\) × 1 ( \(\displaystyle \left( {\frac{2}{3}x} \right)\) + 1 )
= 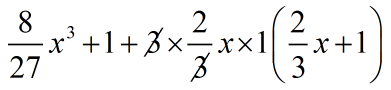
= \(\displaystyle \frac{8}{{27}}{{x}^{3}}\,+\,1+\,2x\,\times \,\left( {\frac{2}{3}x\,+\,1} \right)\)
= \(\displaystyle \frac{8}{{27}}{{x}^{3}}\,+\,1+\,\frac{4}{3}{{x}^{2}}\,+\,2x\)
So, answer = \(\displaystyle \frac{8}{{27}}{{x}^{3}}\,+\,1+\,\frac{4}{3}{{x}^{2}}\,+\,2x\)
(ii) ( 3a - 2b )3
Solution :
We have ( 3a - 2b )3
[ using identity = ( x - y )3 = x3 - y3 - 3xy ( x - y ) ]
( 3a - 2b )3
= ( 3a )3 - ( 2b )3 - 3 × 3a × 2b ( 3a - 2b )
= 27a3 - 8b3 - 18ab ( 3a - 2b )
= 27a3 - 8b3 - 54a2b + 36ab2
So, answer = 27a3 - 8b3 - 54a2b + 36ab2
Question 16. Evaluate :
(i) ( 95 )3
Solution :
We have ( 95 )3
( 100 - 5 )3
[ using identity = ( x - y )3 = x3 - y3 - 3xy ( x - y ) ]
( 100 - 5 )3
= ( 100 )3 - ( 5 )3 - 3 × 100 × 5 ( 100 - 5 )
= 1000000 - 125 - 1500 ( 100 - 5 )
= 1000000 - 125 - 150000 + 7500
= 1007500 - 150125
= 857375
So, answer = 857375
(ii) ( 0.2 )3 - ( 0. 3 )3 + ( 0.1 )3
Solution :
We have ( 0.2 )3 - ( 0. 3 )3 + ( 0.1 )3
= 0.008 - 0.0027 + 0.001
= 0.009 - 0.027
= 0.018
So, answer = 0.018
Question 17. Factorize :
(i) 125a3 + b3 + 64c3 - 60abc
Solution :
We have 125a3 + b3 + 64c3 - 60abc
[ using identity = x3 + y3 + z3 - 3xyz = ( x + y + z ) ( x2 + y2 + z2 - xy - yz - zx ) ]
125a3 + b3 + 64c3 - 60abc
= ( 5a + b + 4c ) ( 5a2 + b2 + 4c2 - 5a × b + b × -4c - 4c × 5a )
= ( 5a + b + 4c ) ( 25a2 + b2 - 5ab - 4bc - 20ca )
So, answer = ( 5a + b + 4c ) ( 25a2 + b2 - 5ab - 4bc - 20ca )
(ii) 216 + 27b3 + 8c3 - 108bc
We have 216 + 27b3 + 8c3 - 108bc
[ using identity = x3 + y3 + z3 - 3xyz = ( x + y + z ) ( x2 + y2 + z2 - xy - yz - zx ) ]
216 + 27b3 + 8c3 - 108bc
= ( 6 + 3b + 2c ) ( 62 + 3b2 + 2c2 - 6 × 3b - 3b × 2c - 2c × 6 )
= ( 6 + 3b + 2c ) ( 36 + 9b2 + 4c2 - 18b - 6bc - 12c )
So, answer = ( 6 + 3b + 2c ) ( 36 + 9b2 + 4c2 - 18b - 6bc - 12c )
Question 18. Find the product :
(i) ( x - 2y + 3 ) ( x2 + 4y2 + 2xy - 3x + 6y + 9 )
We have ( x - 2y + 3 ) ( x2 + 4y2 + 2xy - 3x + 6y + 9 )
Let, First bracket = ( x - 2y + 3 )
Second bracket = ( x2 + 4y2 + 2xy - 3x + 6y + 9 )
[ we have multiply to the first bracket by the second bracket ]
( x - 2y + 3 ) ( x2 + 4y2 + 2xy - 3x + 6y + 9 )
= x ( x2 + 4y2 + 2xy - 3x + 6y + 9 ) - 2y ( x2 + 4y2 + 2xy - 3x + 6y + 9 ) + 3 ( x2 + 4y2 + 2xy - 3x + 6y + 9 )
= x3 + 4xy2 + 2x2y - 3x2 + 6xy + 9x - 2x2y - 8y3 - 4xy2 + 6xy - 12y2 - 18y + 3x2 + 12y2 + 6xy - 9x + 18y + 27
= x3 + 6xy + 6xy + 6xy - 8y3 + 27
= x3 + 18xy - 8y3 + 27
= x3 - 8y3 + 27 + 18xy
So, answer = x3 - 8y3 + 27 + 18xy
Question 19. Write the following in the expanded form :
(i) ( 2a - 3b - c )2
Solution :
We have ( 2a - 3b - c )2
[ using identity = ( x + y + z )2 = ( x2 + y2 + z2 + 2xy + 2yz + 2zx ) ]
( 2a - 3b - c )2
= ( 2a )2 + ( -3b )2 + ( c )2 + 2 × 2a × ( -3b ) + 2 × ( -3b ) × ( -c ) + 2 × ( -c ) × 2a
= 4a2 + 9b2 + c2 - 12ab + 6bc - 4ca
So, answer = 4a2 + 9b2 + c2 - 12ab + 6bc - 4ca
Question 20. Simplify each of the following :
(i) ( 4x + 2y )3 + ( 4x - 2y )3
Solution :
We have ( 4x + 2y )3 + ( 4x - 2y )3
[ using identity = ( x + y )3 = x3 + y3 + 3xy ( x + y ) and ( x - y )3 = x3 - y3 - 3xy ( x - y ) ]
( 4x + 2y )3 + ( 4x - 2y )3
= [ { ( 4x )3 + ( 2y )3 + 3 × 4x × 2y ( 4x + 2y ) } + { ( 4x )3 - ( 2y )3 - 3 × 4x × 2y ( 4x - 2y ) } ]
= 64x3 + 8y3 + 24xy ( 4x + 2y ) + 64x3 - 8y3 - 24xy ( 4x - 2y )
= 64x3 + 8y3 + 96x2y + 48xy2 + 64x3 - 8y3 - 96x2y + 48xy2
= 64x3 + 64x3+ 48xy2 + 48xy2
= 128x3 + 96xy2
So, answer = 128x3 + 96xy2
(ii) 175 × 175 + 2 × 174 × 25 + 25 × 25
Solution :
Given 175 × 175 + 2 × 175 × 25 + 25 × 25
Multiply all these
= 30625 + 8750 + 625
= 40000
S0, answer = 40000 .
NCERT Class 9 Maths Chapter 2 Polynomials :
- NCERT Class 9 Maths Chapter 2 Polynomials Exercise 2.1
- NCERT Class 9 Maths Chapter 2 Polynomials Exercise 2.2
- NCERT Class 9 Maths Chapter 2 Polynomials Exercise 2.3
- NCERT Class 9 Maths Chapter 2 Polynomials Exercise 2.4
- NCERT Class 9 Maths Chapter 2 Polynomials Exercise 2.5
- Extra Questions Class 9 Maths Chapter 2 Polynomials