NCERT Solutions Class 9 Maths Chapter 2 Polynomials Exercise 2.3

 

Introduction:

In this exercise/article we will learn about Polynomials. Remainder theorem, is it not a simple way to find the remainder obtained on dividing a polynomial by a linear polynomial? We will now generalise this fact in the form of the following theorem. We will also show you why the theorem is true, by giving you a proof of the theorem. 

 

NCERT Class 9 Maths Chapter 2 Polynomials :

• NCERT Class 9 Maths Chapter 2 Polynomials Exercise 2.1
• NCERT Class 9 Maths Chapter 2 Polynomials Exercise 2.2
• NCERT Class 9 Maths Chapter 2 Polynomials Exercise 2.3
• NCERT Class 9 Maths Chapter 2 Polynomials Exercise 2.4
• NCERT Class 9 Maths Chapter 2 Polynomials Exercise 2.5
• Extra Questions Class 9 Maths Chapter 2 Polynomials

Class 9 Maths Exercise 2.3 (Page-40)

Q1. Find the remainder when x³ + 3x² + 3x + 1 is divided by

(i) x + 1

Solution :

Let p(x) = x³ + 3x² + 3x + 1

So, x + 1 = 0

x = - 1

Using remainder theorem,

when p(x) = x³ + 3x² + 3x + 1

than p ( - 1 ) = ( - 1 )³ + 3( - 1 )² + 3 × - 1 + 1

= - 1 + 3 × 1 - 3 + 1

= - 1 + 3 - 3 + 1    [ because - 1 + 1 = 0 and 3 - 3 = 0 ]

= 0

So, remainder = 0.

(ii) x - \(\displaystyle \frac{1}{2}\)

Solution :

Let p(x) = x³ + 3x² + 3x + 1

So, x - \(\displaystyle \frac{1}{2}\) = 0

x = \(\displaystyle \frac{1}{2}\)

Using remainder theorem,

When p(x) = x³ + 3x² + 3x + 1

than p( \(\displaystyle \frac{1}{2}\) ) = ( \(\displaystyle \frac{1}{2}\) )³ + 3( \(\displaystyle \frac{1}{2}\) )² + 3 × \(\displaystyle \frac{1}{2}\) + 1

= \(\displaystyle \frac{1}{8}\) + 3 × \(\displaystyle \frac{1}{4}\) + \(\displaystyle \frac{3}{2}\) + 1

= \(\displaystyle \frac{1}{8}\) + \(\displaystyle \frac{3}{4}\) + \(\displaystyle \frac{3}{2}\) + 1

= \(\displaystyle \frac{{1\,+\,6\,+\,12\,+\,8}}{8}\)

= \(\displaystyle \frac{{27}}{8}\)

So, remainder = \(\displaystyle \frac{{27}}{8}\) .

(iii) x

Solution :

Let p(x) = x³ + 3x² + 3x + 1

So, x = 0

x = 0

Using remainder theorem,

when p(x) = x³ + 3x² + 3x + 1

than p( 0 ) = ( 0 )³ + 3( 0 )² + 3 × 0 + 1

= ( 0 )³ + 3 × ( 0 )² + 0 + 1

= 0 + 3 × 0 + 1

= 0 × 0 + 1

= 1

So, remainder = 1 .

(iv) x + \(\displaystyle \pi \)

Solution :

Let p(x) = x³ + 3x² + 3x + 1

So, x + \(\displaystyle \pi \) = 0

x = - \(\displaystyle \pi \)

Using remainder theorem,

when p(x) = x³ + 3x² + 3x + 1

than p( - \(\displaystyle \pi \) ) = ( - \(\displaystyle \pi \) )³ + 3( - \(\displaystyle \pi \) )² + 3 × - \(\displaystyle \pi \) + 1

= - \(\displaystyle \pi \)³ + 3 × \(\displaystyle \pi \)² - 3\(\displaystyle \pi \) + 1

= - \(\displaystyle \pi \)³ + 3 - \(\displaystyle \pi \)² - 3\(\displaystyle \pi \) + 1

So, remainder = - \(\displaystyle \pi \)³ + 3 - \(\displaystyle \pi \)² - 3\(\displaystyle \pi \) + 1

(v) 5 + 2x

Solution :

Let p(x) = x³ + 3x² + 3x + 1

So, 5 + 2x = 0

x = \(\displaystyle -\,\frac{5}{2}\)

Using remainder theorem,

when p(x) = x³ + 3x² + 3x + 1

than p( \(\displaystyle -\,\frac{5}{2}\) ) = ( \(\displaystyle -\,\frac{5}{2}\) )³ + 3(\(\displaystyle -\,\frac{5}{2}\) )² + 3 × \(\displaystyle -\,\frac{5}{2}\) + 1

= \(\displaystyle -\,\frac{{125}}{8}\,+\,3\,\times \,\frac{{25}}{4}\,-\,\frac{{15}}{2}\,+\,1\)

= \(\displaystyle -\,\frac{{125}}{8}\,+\,\frac{{75}}{4}\,-\,\frac{{15}}{2}\,+\,1\)

= \(\displaystyle \frac{{-\,125\,+\,150\,-\,60\,+\,8}}{8}\)

= \(\displaystyle \frac{{-\,185\,+\,158}}{8}\)

= \(\displaystyle -\,\frac{{27}}{8}\)

So, remainder = \(\displaystyle -\,\frac{{27}}{8}\) .

 

NCERT Solutions Class 9 Maths

 

Q2. Find the remainder when x³ - ax² + 6x - a is divided by x - a .

Solution :

Let p(x) = x³ - ax² + 6x - a

So, x - a = 0

= x = a

Using remainder theorem,

when p(x) = x³ - ax² + 6x - a

than p( a ) = ( a )³ - a( a )² + 6 × a - a

= a³ - a × a² + 6a - a

= a³ - a³ + 6a - a        [ because a³ - a³ = 0 ]

= 6a - a

= 5a

So, remainder = 5a .

Q3. Check whether 7 + 3x is a factor of 3x³ + 7x.

Solution :

Let p(x) = 3x³ + 7x

So, 7 + 3x = 0

3x = - 7

x = \(\displaystyle -\,\frac{7}{3}\)

Using remainder theorem,

when p(x) = 3x³ + 7x

than p( \(\displaystyle -\,\frac{7}{3}\) ) = 3x³ + 7x

= 3 ( \(\displaystyle -\,\frac{7}{3}\) )³ + 7 × \(\displaystyle -\,\frac{7}{3}\)

= \(\displaystyle 3\,\times \,-\,\frac{{343}}{{27}}\,-\,\frac{{49}}{3}\)

=

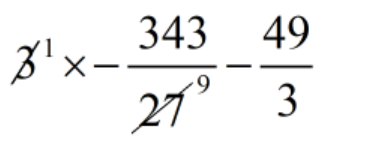
= \(\displaystyle -\,\frac{{343}}{9}\,-\,\frac{{49}}{3}\)

= \(\displaystyle \frac{{-\,343\,-\,147}}{9}\)

= \(\displaystyle -\,\frac{{490}}{9}\)

So, 7 + 3x is not a factor of 3x³ + 7x .

 

NCERT Class 9 Maths Chapter 2 Polynomials :

• NCERT Class 9 Maths Chapter 2 Polynomials Exercise 2.1
• NCERT Class 9 Maths Chapter 2 Polynomials Exercise 2.2
• NCERT Class 9 Maths Chapter 2 Polynomials Exercise 2.3
• NCERT Class 9 Maths Chapter 2 Polynomials Exercise 2.4
• NCERT Class 9 Maths Chapter 2 Polynomials Exercise 2.5