NCERT Solutions Class 8 Maths Chapter 11 Mensuration Exercise 11.4
Introduction :
Amount of space occupied by a three dimensional object is called its volume. We will use cubic units to find the volume of a solid. To find the volume of a cuboid we should know about it's length, breadth and height.(volume of cuboid = area of base x height). The cube is a special case of a cuboid where it's length, breadth and height is equal. Just like cuboid, cylinder has got a top and a base which are congruent and parallel to each other. It's lateral surface is also perpendicular to base just like cuboid.
NCERT Class 9 Maths Chapter 5 Introduction to Euclid's Geometry :
- Class 8 Maths Chapter 11 Mensuration Exercise 11.1
- Class 8 Maths Chapter 11 Mensuration Exercise 11.2
- Class 8 Maths Chapter 11 Mensuration Exercise 11.3
- Class 8 Maths Chapter 11 Mensuration Exercise 11.4
Class 8 Maths Mensuration Exercise-11.4
Q1. Given a cylindrical tank, in which situation will you find the surface area and in which situation volume.
(a) To find how much it can hold.
(b) Number of cement bags required to plaster it.
(c) To find the number of smaller tanks that can be filled with water from it.

Solution:
a)In this situation, we will find volume.
b) In this situation, we will find surface area.
c) In this situation, we will find volume.
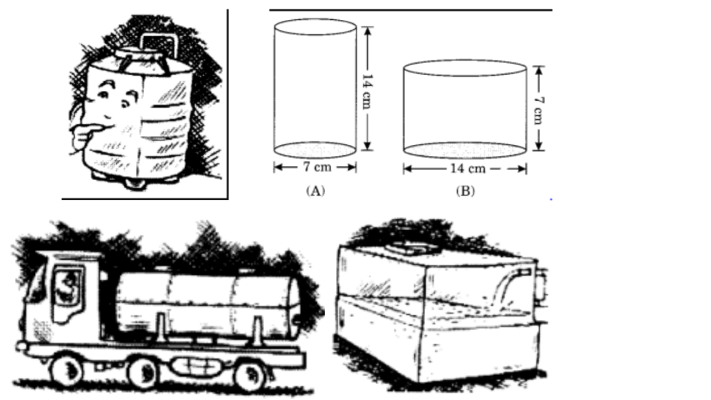
Q2. Diameter of cylinder A is 7 cm, and the height is 14 cm. Diameter of cylinder B is 14 cm and height is 7 cm. Without doing any calculations can you suggest whose volume is greater? Verify it by finding the volume of both the cylinders. Check whether the cylinder with greater volume also has greater surface area?

Solution:
Given: diameter of cylinder A = 7cm
Height of cylinder A = 14cm
diameter of cylinder B = 14cm
Height of cylinder B = 7cm
Cylinder B has greater volume.
verification,
Volume of cylinder A = \(\displaystyle \pi {{r}^{2}}h\)
\(\displaystyle \begin{array}{l}=\,\frac{{22}}{7}\times {{(3.5)}^{2}}\times \,14(r=\frac{d}{2})\\=\,\frac{{22}}{7}\times (3.5)\times (3.5)\,\times \,14\\=22\times (0.5)\times (3.5)\,\times \,14\\=11\times (3.5)\,\times \,14\\=\,38.5\,\times \,14\\=\,539c{{m}^{3}}\end{array}\)
Volume of cylinder B = \(\displaystyle \pi {{r}^{2}}h\)
\(\displaystyle \begin{array}{l}=\,\frac{{22}}{7}\times {{(7)}^{2}}\times \,7(r=\frac{d}{2})\\=\,\frac{{22}}{7}\times (7)\times (7)\,\times \,7\\=22\times \,49\\=\,1078c{{m}^{3}}\end{array}\)
Surface Area of cylinder A = \(\displaystyle 2\pi r(h+r)\)
\(\displaystyle \begin{array}{l}=2\times \frac{{22}}{7}\times 3.5(14+3.5)\\=2\times \frac{{22}}{7}\times 3.5(17.5)\\=\,2\times 22\times 0.5(17.5)\\=\,2\,\times 11\times 17.5\\=\,22\,\times 17.5\\=\,385c{{m}^{2}}\end{array}\)
Surface Area of cylinder B = \(\displaystyle 2\pi r(h+r)\)
\(\displaystyle \begin{array}{l}=2\times \frac{{22}}{7}\times 7(7+7)\\=2\times 22\times (14)\\=\,44\times 14\\=\,616\,c{{m}^{2}}\end{array}\)
so, from the above solution the cylinder with greater volume has also the greater surface area that is cylinder B.
Q3. Find the height of a cuboid whose base area is 180\(\displaystyle c{{m}^{2}}\) and volume is 900 \(\displaystyle c{{m}^{3}}\)?
Solution:
Given: base area of cuboid= 180\(\displaystyle c{{m}^{2}}\)
volume of cuboid= 900\(\displaystyle c{{m}^{3}}\)
as the base area = l\(\displaystyle \times \)b = 180\(\displaystyle c{{m}^{2}}\)
so,
Volume of cuboid = l\(\displaystyle \times \) b \(\displaystyle \times \) h
\(\displaystyle \begin{array}{l}900=\,180\,\times h\\h\,=\,\frac{{900}}{{180}}\\h\,=\,5cm\end{array}\)
hence, the height of cuboid is 5cm.
Q4. A cuboid is of dimensions 60 cm × 54 cm × 30 cm. How many small cubes with side 6 cm can be placed in the given cuboid?
Solution:
Given: length of cuboid=60cm
breadth of cuboid = 54cm
height of cuboid = 30cm
Side of cube = 6cm
Volume of cuboid = l\(\displaystyle \times \) b \(\displaystyle \times \) h
\(\displaystyle \begin{array}{l}=60\times 54\times 30\\=\,3240\times 30\\=\,97200c{{m}^{3}}\end{array}\)
\(\displaystyle \begin{array}{l}volume\,of\,cube\,=\,side\times \,side\,\times side\\=\,6\times \,6\times \,6\\=36\times \,6\\=216c{{m}^{3}}\end{array}\)
Number of small cubes = \(\displaystyle \frac{{volume\,of\,cuboid}}{{volume\,of\,cube}}\)
\(\displaystyle =\,\frac{{97200}}{\begin{array}{l}216\\=\,450\end{array}}\)
hence, 450 small cubes can be placed in the given cuboid.
Q5. Find the height of the cylinder whose volume is 1.54\(\displaystyle {{m}^{3}}\) and the diameter of the base is 140 cm.
Solution:
Given: volume of cylinder = 1.54\(\displaystyle {{m}^{3}}\)
diameter of cylinder = 140cm
Volume of cylinder = \(\displaystyle \pi {{r}^{2}}h\)
\(\displaystyle \begin{array}{l}1.54\,=\,\frac{{22}}{7}\times {{(\frac{{70}}{{100}})}^{2}}\times h\,(r=\frac{d}{2})\\1.54\,=\,\frac{{22}}{7}\times (\frac{{70}}{{100}})\times (\frac{{70}}{{100}})\times h\,\\1.54\,=\,22\times (\frac{{10}}{{100}})\times (\frac{{70}}{{100}})\times h\,\\1.54\,=\,22\times (\frac{7}{{100}})\times h\,\\\frac{{154}}{{100}}\times \frac{{100}}{7}\,=\,22\,\times h\,\\\frac{{154}}{7}=\,22\times h\,\\22\,=\,22\times h\,\\h=\,\frac{{22}}{{22}}\\h\,=\,1\end{array}\)
hence, the height of cylinder is 100cm 0r 1m.
Q6. A milk tank is in the form of a cylinder whose radius is 1.5 m and length is 7 m. Find the quantity of milk in litres that can be stored in the tank.

Solution:
Given: radius of tank = 1.5m
height of tank = 7m
\(\displaystyle \begin{array}{l}volume\,of\,\tan k\,=\,\pi {{r}^{2}}h\\=\,\frac{{22}}{7}\times {{(1.5)}^{2}}\,\times \,7\\=22\times (1.5)\times (1.5)\,\\=\,22\,\times \,(2.25)\\=\,49.5{{m}^{3}}\end{array}\)
converting 49.5 \(\displaystyle {{m}^{3}}\) into litres
= 49.5 \(\displaystyle \times \) 1000 (1 \(\displaystyle {{m}^{3}}\) = 1000litres)
= 49500litres
Hence, 49500 litres of milk can be stored in the tank.
Q7. If each edge of a cube is doubled,
(i) how many times will it be surface area increase?
(ii) how many times will its volume increase?
Solution:
i) Let the edge be = l
If we double the edge then it will be = 2l
now,
Surface area of cube = 6 l2
= 6( 2l)2
= 6 × 4l2
Here, the number 4 indicates that if we double the edge of cube then it's surface area will increase 4 times.
ii) As the volume of cube is = l3
If we double the edge then it will be = 2l
now,
Volume of cube = l3
= (2l)3
= 8 l3
Here, the number 8 indicates that if we double the edge of cube then it's volume will increase 8 times.
Q8. Water is pouring into a cuboidal reservoir at the rate of 60 litres per minute. If the volume of the reservoir is 108 m3 , find the number of hours it will take to fill the reservoir?

Solution:
Given: volume of reservoir = 108m3
rate of flowing water = 60l per minute
Number of hours =\(\displaystyle \frac{{volume\,of\,reservoir}}{{rate\,of\,flowing\,water}}\)
\(\displaystyle =\,\frac{{108\times 1000}}{{60\times 60}}\,\frac{{(As\,1{{m}^{3}}\,=\,1000l)}}{{(1hour\,=\,60\,\min utes)}}\)
\(\displaystyle \begin{array}{l}=\,\frac{{108\times 1000}}{{3600}}\,\\=\,\frac{{108\times 10}}{{36}}\,\\=\,\frac{{1080}}{{36}}\,\\=\,30\end{array}\)
so, it will take 30 hours to fill the reservoir.
NCERT Class 9 Maths Chapter 5 Introduction to Euclid's Geometry :
