NCERT Solutions Class 8 Maths Chapter 11 Mensuration Exercise 11.1

Introduction :

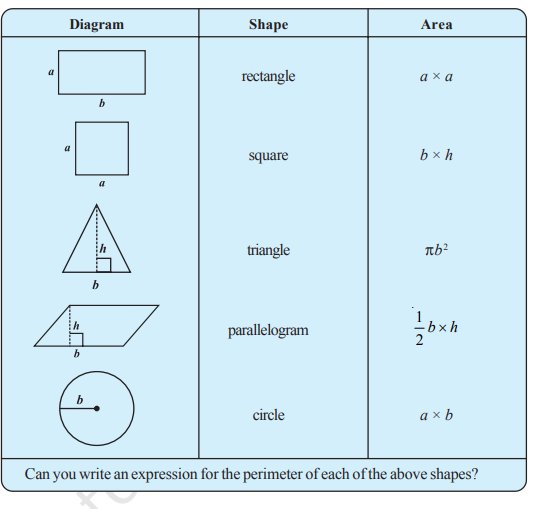
In this exercise we will come to know about Mensuration. solve problems related to perimeter and area of other plane closed figures like quadrilaterals. We will also learn about surface area and volume of solid such as cube, cuboid and cylinder.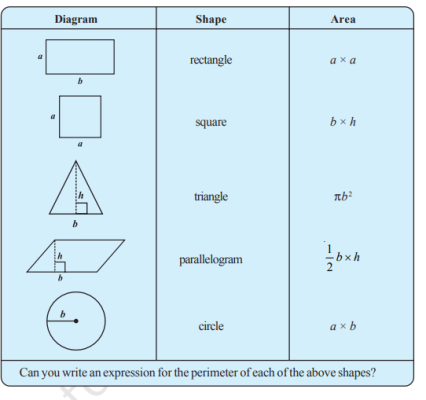

NCERT Class 9 Maths Chapter 5 Introduction to Euclid's Geometry :

Class 8 Maths Mensuration Exercise-11.1

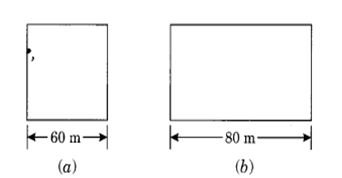
Q1. A square and a rectangular field with measurements given in the figure have the same perimeter. which field has a larger area?

Solution :

According to the question, perimeter of figure (a) = perimeter of figure (b)

Here figure (a) in a square shape and figure (b) in a rectangular shape

So, perimeter of figure (a) = 4 × side

= 4 × 60m = 240m

perimeter of figure (b) = 2(L+B)

=2(80+ B) (∵ L=80 given in the figure)

∵ perimeter of figure (a) = perimeter of figure (b)

240= 2(80+ B)

⇒ 240 = 2×80 + 2B

⇒ 240 = 160 + 2B

⇒ 240-160 = 2B

⇒ 80 = 2B

⇒ 2B = 80

⇒ B = 40m

Now, area of figure (a) = side × side

= 60m × 60m = 3600m²

area of figure (b) = L × B = 80m × 40m = 3200m²

So, area of figure (a) higher than figure (b)

If you not Understand than Click below for step by steps LIVE VIDEO

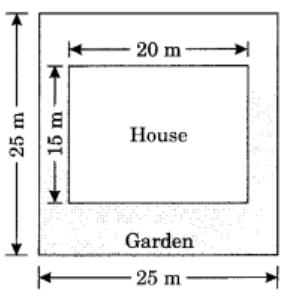
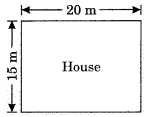
Q2. Mrs Kaushik has a square plot with the measurement as shown in the figure. She wants to construct a house in the middle of the plot. A garden is developed around the house. Find the total cost of developing a garden around the house at the rate of ₹ 55 per m².

Solution :

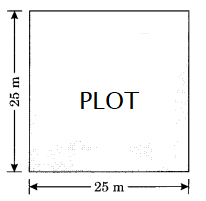
According to the given figure plot in "square shape"

So, Area of plot = side × side

= 25m × 25m = 625m²

House is in rectangular shape

here L=20m and B=15m

So, area of house = L×B =20m×15m=300m²

Now, we need to find the area of Garden

So, area of garden = (area of plot - area of house)

= (625 - 300)m² = 325m²

∵ Cost of developing the garden 1m² = ₹ 55

So, cost of developing the garden 325m² = ₹ 55 × 325 = ₹ 17875

If you not Understand than Click below for step by steps LIVE VIDEO

Q3. The shape of a garden is rectangular in the middle and semi circular at the ends as shows in the diagram. Find the area and the perimeter of the garden [Length of rectangle is 20 - (3.5+3.5) meter].

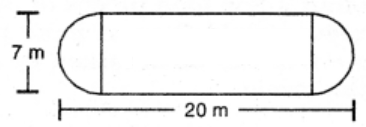
Solution :

According to the given diagram, here 1 rectangle and 2 semi circles

here, Length of rectangle L = 20-(3.5+3.5) = 13m

and Breath of rectangle B = 7m

Radius of semicircle r = 3.5m

So, area of garden = (area of rectangle) + (area of 2 semi circles)

= (L × B) + ( 2 × \(\displaystyle \frac{{\pi {{r}^{2}}}}{2}\) )

= (L × B) + (\(\displaystyle {\pi {{r}^{2}}}\))

= (13 × 7) + { \(\displaystyle \frac{{22}}{7}\times \,{{(3.5)}^{2}}\)}

= \(\displaystyle 91+\frac{{22}}{7}\times \,\frac{7}{2}\times \frac{7}{2}\)

= \(\displaystyle 91+\frac{{77}}{2}\)

= \(\displaystyle \frac{{182+77}}{2}\)

= \(\displaystyle \frac{{259}}{2}\) = 129.5m²

Perimeter of garden = perimeter of 2 semicircle + 2(length of rectangle)

= \(\displaystyle 2(\pi r)\)+2L

=\(\displaystyle 2\times \frac{{22}}{7}\times \frac{7}{2}+2\times 13\)

= 22+26 = 48m.

If you not Understand than Click below for step by steps LIVE VIDEO

Q4. A flooring tile has the shape of a parallelogram whose base is 24 cm and the corresponding height is 10 cm. How many such tiles are required to cover a floor of area 1080 m²? (If required you can split the tiles in whatever way you want to fill up the corners).

Solution:

Given: Base = 24cm

height=10cm

Area of floor=1080 m²

To find: Number of tiles

As the tiles are in shape of parallelogram so,

Area of parallelogram= Base × Height

= 24 × 10

=240cm²

So, the area of 1 tile is 240 cm²

Now, the number of tiles required is

=\(\displaystyle \frac{{Area\,of\,floor}}{{Area\,of\,each\,tile}}\)

=\(\displaystyle \frac{{1080}}{{240}}\) (convert 1080m² into cm²)

= \(\displaystyle \frac{{1080\times 10000}}{{240}}\)

= 4.5 × 10000

= 45,000

So, the number of tiles required is 45,000

If you not Understand than Click below for step by steps LIVE VIDEO

Q5. An ant is moving around a few food pieces of different shapes scattered on the floor. For which food-piece would the ant have to take a longer round? Remember circumference of a circle can be obtained by using the expression c= 2\(\displaystyle \pi \)r,where r is the radius of the circle.

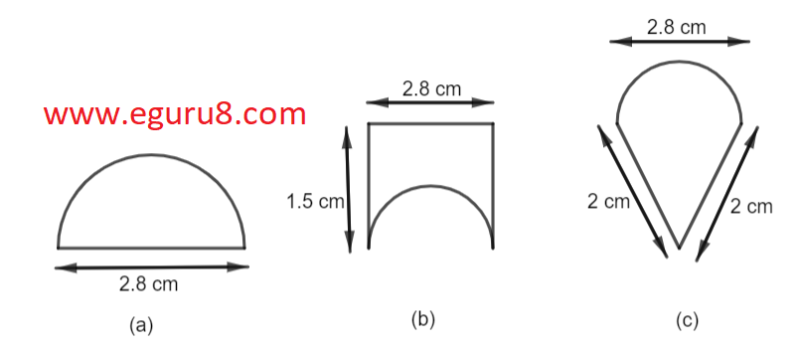
Solution:

According to Question,

a) The first figure is semi-circle so,

Perimeter of semi-circle is =\(\displaystyle \pi \)r+d (d=diameter, r= radius)

=(\(\displaystyle \frac{{22}}{7}\)\(\displaystyle \times \)1.4)+2.8 (r=\(\displaystyle \frac{d}{2}\))

=(22\(\displaystyle \times \)0.2)+2.8

=4.4+2.8

=7.2cm

b)Perimeter of given figure is =\(\displaystyle \pi \)r+d+2S (2S= 2\(\displaystyle \times \)side)

(\(\displaystyle \frac{{22}}{7}\)\(\displaystyle \times \)1.4)+(2.8)+(2\(\displaystyle \times \)1.5)

=(22\(\displaystyle \times \)0.2)+2.8+3.0

=4.4+2.8+3.0

=10.2cm

c) Perimeter of given figure is = \(\displaystyle \pi \)r+ 2S (2S= 2\(\displaystyle \times \)side)

=(\(\displaystyle \frac{{22}}{7}\)\(\displaystyle \times \)1.4)+(2\(\displaystyle \times \)2)

=(22\(\displaystyle \times \)0.2)+4

=4.4+4

=8.4cm

So, from the above observation the ant will have to take the longer round of figure(b) food piece.

If you not Understand than Click below for step by steps LIVE VIDEO

NCERT Class 9 Maths Chapter 5 Introduction to Euclid's Geometry :

NCERT Solutions Class 8 Maths Chapter 11 Mensuration Exercise 11.1

Introduction :

Class 8 Maths Mensuration Exercise-11.1

Leave a Comment Cancel Reply