NCERT Solutions Class 9 Maths Chapter 13 Surface area and Volume Exercise 13.2

Introduction:

In this exercise/article we will learn about Surface Area And Volume. Surface area of a Right Circular Cylinder. Curved surface area and Total surface area of a cylinder. this exercise based only on cylinder of formula. You know π = 227. Here, we will be dealing with only right circular cylinders. So, unless started otherwise, the word cylinder would means a right circular cylinder. In the case of a cylinder, unless started otherwise, 'Radius of a cylinder' will mean 'base radius of the cylinder '.

Class 9 Maths Chapter 13 Surface Area And Volume :

Class 9 Maths Exercise 13.2 (Page-216)

Q1. The curved surface area of a right circular cylinder of height 14 cm is 88 cm². Find the diameter of the base of the cylinder.

Solution :

According to the question,

Given, Curved surface area of cylinder = 88 cm²

Height = 14 cm

So, Curved surface area of cylinder = 2πrh

⇒ 88 = 2 × \(\displaystyle \frac{{22}}{7}\) × r × 14

⇒ 88 = \(\displaystyle \frac{{44}}{7}\) × r × 14

⇒ 88 × 7 = 616r

⇒ 616 = 616r

⇒ \(\displaystyle \frac{{616}}{{616}}\) cm = r

Radius = 1 cm

∴ D = r × 2

⇒ D = 1 cm × 2

⇒ D = 2 cm

∴ Diameter of the base of the cylinder = 2 cm .

Q2. It is required to make a closed cylindrical tank of height 1 m and base diameter 140 cm from a metal sheet. How many square meters of the sheet are required for the same?

Solution :

According to the question,

Radius = \(\displaystyle \frac{d}{2}\)

Radius = \(\displaystyle \frac{{140}}{2}\)

Radius = 70 cm

Given, Radius = 0.7 m [ converted cm to m ]

Height = 1 m

So, Total surface area of cylindrical tank = 2πr ( r + h )

= 2 × \(\displaystyle \frac{{22}}{7}\) × 0.7 ( 0.7 + 1 )

= \(\displaystyle \frac{{44}}{7}\) × 0.7 × 1.7

= \(\displaystyle \frac{{52.36}}{7}\)

= 7.48 m2

∴ 7.48 square meter of the sheet are required .

Q3. A metal pipe is 77 cm long. The inner diameter of a cross section is 4 cm, the outer diameter being 4.4 cm ( see fig. 13.11 ). Find its

(i) inner curved surface area,

(ii) outer curved surface area,

(iii) total surface area.

Solution :

According to the given figure ''cylindrical''

(i) Radius = \(\displaystyle \frac{d}{2}\)

Radius = \(\displaystyle \frac{{4}}{2}\)

Given, Radius = 2 cm

Height/Length = 77

So, Inner curved surface area of pipe = 2πrh

= 2 × \(\displaystyle \frac{{22}}{7}\) × 2 × 77

= \(\displaystyle \frac{{88}}{7}\) × 77

= 968 cm2

∴ Inner curved surface area of pipe = 968 cm2

(ii) Radius = \(\displaystyle \frac{d}{2}\)

Radius = \(\displaystyle \frac{{4.4}}{2}\)

Given, Radius = 2.2 cm

Height/Length = 77

So, Outer curved surface area of pipe = 2πrh

= 2 × \(\displaystyle \frac{{22}}{7}\) × 2.2 × 77

= \(\displaystyle \frac{{44}}{7}\) × 2.2 × 77

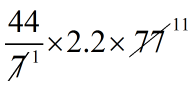
= 44 × 2.2 × 11

= 1064.8 cm2

∴ Outer curved surface area of pipe = 1064.8 cm2

(iii) Let the, inner radius = R

outer radius = r

So, Area of pipe = 2 × π ( R2 - r2 )

= 2 × \(\displaystyle \frac{{22}}{7}\) × ( 2 + 2.2 ) ( 2 - 2.2 ) [ = a2 - b2 = ( a + b ) ( a - b ) ]

= \(\displaystyle \frac{{44}}{7}\) ( 4.2 ) ( 0.2 )

= 44 × 0.2 × 0.6

= 5.28 cm2

Now, Total surface area of pipe = ( 968 + 1064.8 + 5.28 )

= 2038.08 cm2

∴ Total surface area of pipe = 2038.08 cm2 .

Q4. The diameter of a roller is 84 cm and its length is 120 cm. It takes 500 completes revolution to move once over to level a playground. Find the area of the playground in m² .

Solution :

According to the question,

Radius = \(\displaystyle \frac{d}{2}\)

Radius = \(\displaystyle \frac{{84}}{2}\)

Radius = 42 cm

Given, Radius = 0.42 m [ converted cm to m ]

Height/Length = 1.20 m [ converted cm to m ]

So, Curved surface area of roller = 2πrh

= 2 × \(\displaystyle \frac{{22}}{7}\) × 0.42 × 1.20

= \(\displaystyle \frac{{44}}{7}\) × 0.42 × 1.20

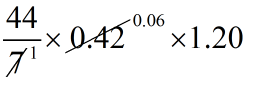
= 44 × 0.06 × 1.20

= 3.168 m2

Area of playground levelled in 1 revolution = 3.168 m2

Now, Area of playground levelled in 500 revolution = 3.168 × 500

= 15840 m2

∴ Area of the playground = 15840 m2 .

Q5. A cylindrical pillar is 50 cm in diameter and 3.5 m in height. Find the cost of painting the curved surface area of the pillar at the rate of Rs 12.50 per m² .

Solution :

According to the question,

Radius = \(\displaystyle \frac{d}{2}\)

Radius = \(\displaystyle \frac{{50}}{2}\)

Radius = 25 cm

Given, Radius = 0.25 m [ converted cm to m ]

Height/Length = 3.5 m

So, Curved surface area of the pillar = 2πrh

= 2 × \(\displaystyle \frac{{22}}{7}\) × 0.25 × 3.5

= \(\displaystyle \frac{{44}}{7}\) × 0.25 × 3.5

= 44 × 0.25 × 0.5

= 5.5 m2

Now, Cost of painting 1 m2 = Rs 12.50 per m2

Cost of painting 5.5 m2 = Rs 12.50 × 5.5

= Rs 68.75

∴ Total cost of painting the cylindrical pillar = Rs 68.75 .

Q7. The inner diameter of a circular well is 3.5 m. It is 10 m deep. Find

(i) its inner curved surface area,

(ii) the cost of plastering this curved surface at the rate of Rs 40 per m2 .

Solution :

According to the question,

Radius = \(\displaystyle \frac{d}{2}\)

Radius = \(\displaystyle \frac{{3.5}}{2}\)

Radius = 1.75 m

(i) Given, Radius = 1.75 m

Height = 10 m

So, Inner curved surface area of a circular well = 2πrh

= 2 × \(\displaystyle \frac{{22}}{7}\) × 1.75 × 10

= \(\displaystyle \frac{{44}}{7}\) × 1.75 × 10

= \(\displaystyle \frac{{440}}{7}\) × 1.75

= \(\displaystyle \frac{{770}}{7}\)

= 110 m2

∴ inner curved surface area of a circular well = 110 m2

(ii) Cost of plastering 1 m2 = Rs 40 per m2

Cost of plastering 110 m2 = Rs 40 × 110

= Rs 4400

∴ Total cost of plastering = Rs 4400 .

Q8. In a hot water heating system, there is a cylindrical pipe of length 28 m and diameter 5 cm. Find the total radiating surface in the system.

Solution :

According to the question,

Radius = \(\displaystyle \frac{d}{2}\)

Radius = \(\displaystyle \frac{{5}}{2}\)

Radius = 2.5 cm

(i) Given, Radius = 0.025 m [ converted cm to m ]

Height/Length = 28 m

So, Total surface area of cylindrical pipe = 2πr ( r + h )

= 2 × \(\displaystyle \frac{{22}}{7}\) × 0.025 ( 0.025 + 28 )

= \(\displaystyle \frac{{44}}{7}\) × 0.025 ( 28.025 )

= \(\displaystyle \frac{{1.1}}{7}\) × 28.025

= \(\displaystyle \frac{{30.8275}}{7}\)

= 4.4 m2

∴ Total radiating surface in the system = 4.4 m2 .

Q9. Find

(i) the lateral or curved surface area of a closed cylindrical petrol storage tank that is 4.2 m in diameter and 4.5 m high .

(ii) how much steel was actually used, if \(\displaystyle \frac{1}{{12}}\) of the steel actually used was wasted in making the tank.

Solution :

According to the question,

Radius = \(\displaystyle \frac{d}{2}\)

Radius = \(\displaystyle \frac{{4.2}}{2}\)

Radius = 2.1 m

(i) Given, Radius = 2.1 m

Height/Length = 4.5 m

So, Curved surface area of a petrol storage tank = 2πrh

= 2 × \(\displaystyle \frac{{22}}{7}\) × 2.1 × 4.5

= \(\displaystyle \frac{{44}}{7}\) × 9.45

= \(\displaystyle \frac{{415.8}}{7}\)

= 59.4 m2

∴ Lateral or curved surface area of a petrol storage tank = 59.4 m2

(ii) Total surface area of a petrol storage tank = 2πr ( r + h )

= 2 × \(\displaystyle \frac{{22}}{7}\) × 2.1 ( 2.1 + 4.5 )

= \(\displaystyle \frac{{44}}{7}\) × 2.1 ( 6.6 )

= \(\displaystyle \frac{{44}}{7}\) × 13.86

= \(\displaystyle \frac{{609.84}}{7}\)

= 87.12 m2

Now, Let steel actually used = x m2

Then, Actually used - waste = used in tank

x - \(\displaystyle \frac{1}{{12}}\) = 87.12 m2

⇒ \(\displaystyle \frac{{11}}{{12}}x\) = 87.12

⇒ 11x = 87.12 × 12

⇒ 11x = 1045.44

⇒ x = \(\displaystyle \frac{{1045.44~}}{{11}}\)

⇒ 95.04 m2

∴ Total surface area of a petrol storage tank = 95.04 m2 .

Q10. In fig. 13.12, you see the frame of a lampshade. It is to be covered with a decorative cloth. The frame has a base diameter of 20 cm and height of 30 cm. A margin of 2.5 cm is to be given for folding it over the top and bottom of the frame. Find how much cloth is required for covering the lampshade.

Solution :

According to the given figure ''cylindrical''

Radius = \(\displaystyle \frac{d}{2}\)

Radius = \(\displaystyle \frac{{20}}{2}\)

Radius = 10 cm

(i) Given, Radius = 10 cm

Height = 30 cm

So, The height of margin for folding to cover, including the top and bottom height = ( 30 + 2.5 + 2.5 )

= 30 + 5

= 35 cm

Now, Total area of cloth for covering the lampshade = 2πrh

= 2 × \(\displaystyle \frac{{22}}{7}\) × 10 × 35

= \(\displaystyle \frac{{44}}{7}\) × 350

= \(\displaystyle \frac{{15400}}{7}\)

= 2200 cm2

∴ 2200 cm2 cloth is required for covering the lampshade .

Q11. The students of a Vidyalaya were asked to participate in a competition for making and decorating penholders in the shape of a cylinder with a base, using cardboard. Each penholder was to be of radius 3 cm and height 10.5 cm. The vidyalaya was to supply the competitors with cardboard. If there were 35 competitors, how much cardboard was required to be bought for the competition?

Solution :

According to the question,

Given, Radius = 3 cm

Height = 10.5 cm

So, Area of penholder and including top

= ( curved surface area ) + ( Base area )

2πrh + πr2

= 2 × π × 3 × 10.5 + π × ( 3 )2

= 63π + 9π cm2

= 72π cm2

Now, The area of cardboard of 35 penholder = 35 × 72π

= 35 × 72 × \(\displaystyle \frac{{22}}{7}\)

= 2520 × \(\displaystyle \frac{{22}}{7}\)

= \(\displaystyle \frac{{55440}}{7}\)

= 7920 cm2

∴ 7920 cm2 cardboard has required to be bought for the competition .

Class 9 Maths Chapter 13 Surface Area And Volume :

NCERT Solutions Class 9 Maths Chapter 13 Surface Area And Volume Exercise 13.2

NCERT Solutions Class 9 Maths Chapter 13 Surface area and Volume Exercise 13.2

Introduction:

Class 9 Maths Exercise 13.2 (Page-216)

Leave a Comment Cancel Reply