NCERT Solutions Class 8 Maths Chapter 7 Cubes and Cube Roots Exercise 7.2
Introduction :
In this exercise/article we will learn about cubes and cube roots. Cube is 3d picture of square and you will find the cubes root of a cube number in this exercise and find cube and cube roots through prime factorisation method . The symbol is cube root \(\displaystyle \sqrt[3]{{}}\) .
NCERT Solutions Class 8 Maths Chapter 7 Cubes and Cube Roots :
- NCERT Class 8 Maths Chapter 7 Cubes and Cube Roots Exercise 7.1
- NCERT Class 8 Maths Chapter 7 Cubes and Cube Roots Exercise 7.2
Class 8 Maths Exercise 7.2 (Page-116)
Q1. Find the cube root of each of the following numbers by the prime factorisation method.
(i) 64
Solution :
Given, 64

= \(\displaystyle \sqrt[3]{{64}}\)
= \(\displaystyle \sqrt[3]{{2\times 2\times 2\times 2\times 2\times 2}}\)
= \(\displaystyle \sqrt[3]{{{{2}^{3}}\times {{2}^{3}}}}\)
= 2 × 2
= 4
Hence, the cube root of 64 = 4
(ii) 512
Solution :
Given, 512
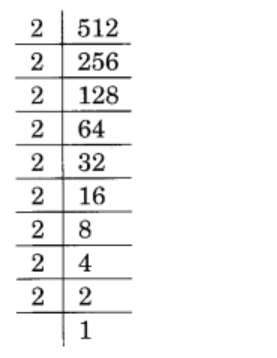
= \(\displaystyle \sqrt[3]{{512}}\)
= \(\displaystyle \sqrt[3]{{2\times 2\times 2\times 2\times 2\times 2\times 2\times 2\times 2}}\)
= \(\displaystyle \sqrt[3]{{{{2}^{3}}\times {{2}^{3}}\times {{2}^{3}}}}\)
= 2 × 2 × 2
= 8
Hence, the cube root of 512 = 8
(iii) 10648
Solution :
Given, 10648

= \(\displaystyle \sqrt[3]{{10648}}\)
= \(\displaystyle \sqrt[3]{{2\times 2\times 2\times 11\times 11\times 11}}\)
= \(\displaystyle \sqrt[3]{{{{2}^{3}}\times {{{11}}^{3}}}}\)
= 2 × 11
= 22
Hence, the cube root of 10648 = 22
(iv) 27000
Solution :
Given, 27000

= \(\displaystyle \sqrt[3]{{27000}}\)
= \(\displaystyle \sqrt[3]{{2\times 2\times 2\times 3\times 3\times 3\times 5\times 5\times 5}}\)
= \(\displaystyle \sqrt[3]{{{{2}^{3}}\times {{3}^{3}}\times {{5}^{3}}}}\)
= 2 × 3 × 5
= 30
Hence, the cube root of 27000 = 30
(v) 15625
Solution :
Given, 15625

= \(\displaystyle \sqrt[3]{{15625}}\)
= \(\displaystyle \sqrt[3]{{5\times 5\times 5\times 5\times 5\times 5}}\)
= \(\displaystyle \sqrt[3]{{{{5}^{3}}\times {{5}^{3}}}}\)
= 5 × 5
= 25
Hence, the cube root of 15625 = 25
(vi) 13824
Solution :
Given, 13824

= \(\displaystyle \sqrt[3]{{13824}}\)
= \(\displaystyle \sqrt[3]{{2\,\times \,2\,\times \,2\,\times \,2\,\times \,2\,\times \,2\,\times \,2\,\times \,2\,\times \,2\,\times \,3\,\times \,3\,\times \,3}}\,\)
= \(\displaystyle \sqrt{{{{2}^{3}}\,\times \,{{2}^{3}}\,\times {{2}^{3}}\,\times {{3}^{3}}}}\)
= 2 × 2 × 2 × 3
= 24
Hence, the cube root of 13824 = 24
(vii) 110592
Solution :
Given, 110592

= \(\displaystyle \sqrt[3]{{110592}}\)
= \(\displaystyle \sqrt[3]{{2\,\times \,2\,\times \,2\,\times \,2\,\times \,2\,\times \,2\,\times \,2\,\times \,2\,\times \,2\,\times \,2\,\times \,2\,\times \,2\,\times \,3\,\times \,3\,\times \,3}}\,\)
= \(\displaystyle \sqrt{{{{2}^{3}}\,\times \,{{2}^{3}}\,\times {{2}^{3}}\,\times {{2}^{3}}\,\times {{3}^{3}}}}\)
= 2 × 2 × 2 × 2 × 3
= 48
Hence, the cube root of 110592 = 48
(viii) 46656
Solution :
Given, 46656

NCERT Solutions Class 8 Maths Chapter 7 Cubes and Cube Roots :