NCERT Solutions Class 8 Maths Chapter 14 Factorisation Exercise 14.1

 

Introduction :

In this exercise we will learn about Factorisation. What is Factorisation? When we Factorise an algebraic expression, we write it as a product of factors. These factors may be numbers, algebraic variable or algebraic expressions.  Factor of algebraic expressions. Method of common factors. Factorisation by regrouping terms.

 

NCERT Class 9 Maths Chapter 5 Introduction to Euclid's Geometry :

• NCERT Class 8 Maths Understanding Factorisation Exercise 14.1
• NCERT Class 8 Maths Understanding Factorisation Exercise 14.2
• NCERT Class 8 Maths Understanding Factorisation Exercise 14.3
• NCERT Class 8 Maths Understanding Factorisation Exercise 14.4

NCERT Class 8 Maths Exercise 14.1 (Page-220)

Q1. Find the common factors of the given terms.

(i) 12x, 36

Solutions:

 

(ii) 2y, 22xy

Solutions:

 

(iii) \(14pq,28{{p}^{2}}{{q}^{2}}\)

Solutions:

 

(iv).\(2x,3{{x}^{2}},4\)

Solutions:

 

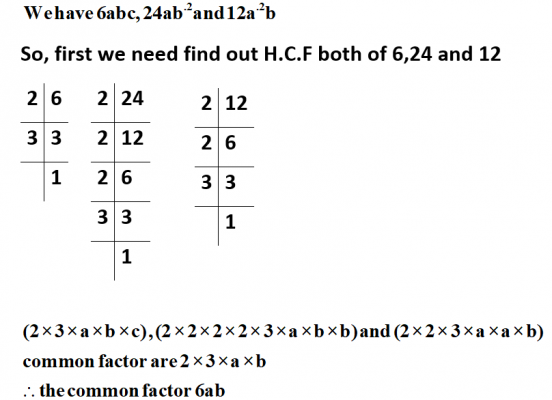
(v).\(6abc,24a{{b}^{2}},12{{a}^{2}}b\)

Solutions:

 

(vi) \(\displaystyle 16{{x}^{3}},-4{{x}^{2}},32x\)

Solutions:

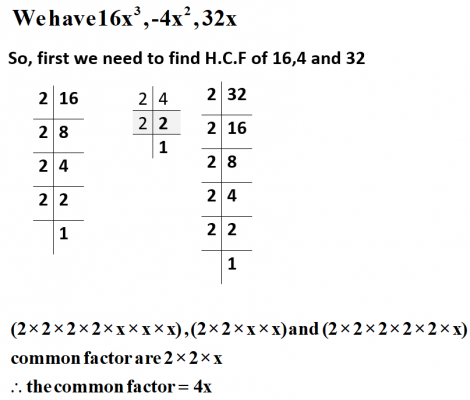
(vii) 10pq, 20qr, 30rp

Solutions:

 

Q2. Factorise the following expressions.

(i) 7x - 42

solution:

we have 7x - 42

we need to factorise this

7x - 42

= 7( x - 6)

∴ The factor of 7x - 42 is 7( x - 6) .

(ii) 6p-12q

solution:

We have 6p-12q

We need to factorise this

6p-12q

= 6(p - 2q)

∴ The factor of 6p-12q is 6(p - 2q) .

(iii) 7a2 + 14a

solution:

We have 7a2 + 14a

we need to factorise this

7a2 + 14a

= 7a(a + 2)

∴ The factor of 7a2 + 14a is 7a(a + 2) .

(iv) -16z + 20z3

solution:

We have -16z +20z3

we need to factorise this 

=-4z ( 4 + 5z2 )

∴ The factor of -16 + 20z3 is -4z(4 + 5z2)

(v) 20l2 m + 30alm

Solution:

We have 20l2 m+ 30alm

we need to factorise this

=10lm ( 2l + 3a )

∴ The factor of 20l2 m+ 30alm is 10lm ( 2l + 3a )

(vi) 5x2 y - 15xy2

solution:

We have 5x2 y - 15xy2

We need to factorise this

=5xy ( x + 3y2 )

∴ The factor of 5x2 y - 15xy2 is 5xy ( x + 3y2 ).

(vii) 10a2 - 15b2 + 20c2

solution:

We have 10a2 - 15b2 + 20c2

we need to factorise this

= 5 ( 2a2 - 3b2 + 4c2 )

∴The factor of 10a2 - 15b2 + 20c2  is 5 ( 2a2 - 3b2 + 4c2 ).

(viii) -4a2+4ab - 4ca

Solution:

We have -4a2+4ab - 4ca

we need to factorise this

= -4×a×a + 4×a×b - 4×c×a

= -4a ( a - b + c )

∴The factor of -4a2+4ab - 4ca is -4a ( a - b + c ) .

(ix) x2yz + xy2z +xyz2

solution:

We have x2yz + xy2z +xyz2

we need to factorise this

= x×x×y×z + x×y×y×z + x×y×z×z

= xyz ( x + y + z )

∴ The factor of x2yz + xy2z +xyz2 is xyz ( x + y + z ).

(x) ax2y +bxy2 +cxyz

Solution:

We have ax2y +bxy2 +cxyz

we need to factorise this

= a×x×x×y + b×x×y×y + c×x×y×z

= xy ( ax + by + cz )

Q3. Factorise.

(i) x2 + xy + 8x + 8y

Solution :

We have  x2 + xy + 8x + 8y  to  factorise

by grouping the terms  

= x ( x + y ) + 8 ( x + y )

= ( x + y ) ( x + 8 )

( ii ) 15xy - 6x + 5y - 2 

Solution :

We have  15xy - 6x + 5y - 2 to factorise

by grouping the terms

= (15xy - 6x ) + ( 5y - 2 ) 

= 3x ( 5y - 2 ) + ( 5y - 2 )

= ( 3x + 1 ) ( 5y - 2 )

( iii ) ax + bx - ay - by

Solution:

we have ax + bx - ay - by to factorise

by grouping the terms 

= x ( a + b ) - y ( a + b )

= ( x - y ) ( a + b ) 

( iv ) 15pq + 15 + 9q + 25p

Solution : 

We have 15pq + 15 + 9q + 25p to factorise

by grouping the terms

= ( 15pq + 25p ) ( 9q + 15 )

= 5p ( 3q + 5 ) + 3 (3q + 5 )

=  ( 3q + 5 ) ( 5p + 3 )

( v ) z - 7 + 7xy -xyz

solution :

 We have z - 7 + 7xy -xyz  to factorise 

by grouping the terms

= ( -xyz + 7xy ) ( z - 7 )

= - xy ( z - 7 ) + 1 ( z - 7 )

= ( -xy + 1 ) ( z - 7 )

 

 

 

 

 

NCERT Class 9 Maths Chapter 5 Introduction to Euclid's Geometry :

• NCERT Class 8 Maths Understanding Factorisation Exercise 14.1
• NCERT Class 8 Maths Understanding Factorisation Exercise 14.2
• NCERT Class 8 Maths Understanding Factorisation Exercise 14.3